CLASS-6
CORRESPONDING ANGLES
CORRESPONDING ANGLES -
Corresponding angles are a type of angle relationship formed when a transversal intersects two parallel lines. When this occurs, corresponding angles are found on the same side of the transversal and in the same relative position with respect to the parallel lines. Here are the key properties of corresponding angles:-
(1) Definition:-
- Corresponding angles are angles that occupy the same relative position at each intersection on the same side of the transversal.
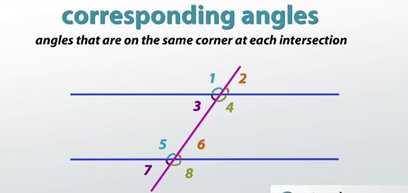
(2) Location:-
- Corresponding angles are found on the same side of the transversal.
(3) Parallel Lines:-
- Corresponding angles are congruent (equal) when the lines intersected by the transversal are parallel.
(4) Symbolically:-
- If two lines are parallel, corresponding angles are denoted as ∠1 ≅ ∠5, ∠2 ≅ ∠6, and so on.
(5) Relationship:-
Corresponding angles have the same measure and exhibit similar geometric characteristics.
In a diagram where a transversal intersects two parallel lines, corresponding angles are typically identified using specific angle markings or letters. For example, if ∠1 and ∠5 are corresponding angles, then they are equal, and the same applies to pairs like ∠2 and ∠6, ∠3 and ∠7, and so forth.
Understanding the concept of corresponding angles is crucial in geometry, particularly when solving problems involving parallel lines and transversals. It is one of the fundamental angle relationships formed in geometric configurations with intersecting lines.