CLASS-6
GEOMETRY - PERPENDICULAR LINES

PERPENDICULAR LINES -

Perpendicular lines are two lines that intersect at a right angle (90 degrees). In other words, the slopes of perpendicular lines are negative reciprocals of each other. If the slope of one line is m, the slope of a line perpendicular to it is −1/m.

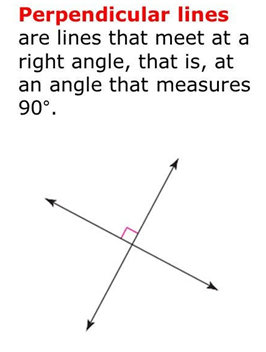
Key properties and concepts related to perpendicular lines include:

1. Right Angle:- The intersection of perpendicular lines forms a right angle, which measures 90 degrees. This 90-degree angle is formed by the two segments of the lines on either side of the point of intersection.
2. Negative Reciprocal Slopes:- If the slope of one line is m, the slope of a line perpendicular to it is −1/m. This relationship ensures that the product of the slopes is -1.
3. Symbol:- The symbol ⊥ is often used to indicate perpendicularity. For example, if line AB is perpendicular to line CD, it is written as AB ⊥ CD.
4. Orthogonality:- In three-dimensional space, the concept of perpendicularity extends to orthogonality. Three lines are said to be mutually perpendicular if each pair of lines forms a set of perpendicular lines.

Perpendicular lines are common in geometry and have practical applications in various fields, such as architecture, engineering, and physics. In architectural drawings, perpendicular lines help create right angles for constructing buildings. In engineering and physics, the concept of perpendicularity is crucial for understanding forces, vectors, and spatial relationships.