CLASS-6
MULTIPLICATION OF ALGEBRAIC EXPRESSION
MULTIPLICATION OF ALGEBRAIC EXPRESSION -
Multiplying algebraic expressions involves applying the distributive property and combining like terms. Here are the steps to multiply two algebraic expressions:
- Distribute:- If you have a term outside parentheses and you need to multiply it by an expression inside the parentheses, you use the distributive property. For example, if you have a (b+c), you distribute a to both b and c to get ab+ac.
- Multiply Like Terms:- After distributing, you may have like terms that can be combined. Like terms are terms that have the same variables raised to the same powers. For example, 3x and 2x are like terms, but 3x and 2x² are not. For example, if you have 3x+2x, you can combine these to get 5x.
- Deal with Exponents:- If there are exponents involved, make sure to apply the rules of exponents. For instance, when you multiply like terms with the same base, you add the exponents. For example, x² ⋅ x³ = x³+² = x⁵.
Here's an example to illustrate these steps:
Let's say you want to multiply (2x+3)(4x−1).
- Distribute the terms: 2x⋅4x + 2x⋅(−1) + 3⋅4x + 3⋅(−1)
This simplifies to: 8x² − 2x + 12x − 3
- Combine like terms: 8x² + 10x − 3
So, (2x+3) (4x−1) = 8x² + 10x − 3.
Remember to always check your work to ensure you have simplified the expression as much as possible!
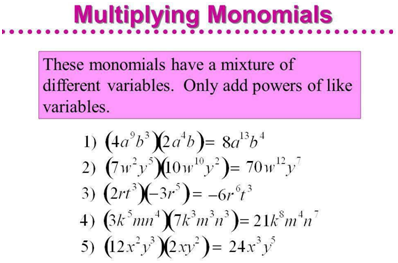
(i) Take note of following points for like terms:-
(a) The coefficients will get multiplied.
(b) The power of the resultant variable will be the addition of the individual powers.
Example.1):- Product of 2x and 3x will be 6x².
Example.2):- Productof 2x, 3x and 4x will be 24x³.

(i) Take note of following points for unlike terms:-
(a)The coefficients will get multiplied.
(b) If all the variables are different then there will be no change in the power of variables.
(c) If some of the variables are same then the respective power of variables will be added.