CLASS-4
DIAGONAL OF A RECTANGLE

DIAGONAL OF A RECTANGLE -

The diagonal of a rectangle is the line segment that connects opposite corners of the rectangle. It is also the longest side of the rectangle and is equal to the square root of the sum of the squares of the length and width of the rectangle.

The diagonal of a rectangle is the line segment that connects opposite corners of the rectangle. To find the length of the diagonal, you can use the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse (the longest side) is equal to the sum of the squares of the lengths of the other two sides.

In a rectangle, the diagonal is the hypotenuse of a right triangle, where the two other sides are the length and the width of the rectangle.

If we let "L" be the length of the rectangle and "W" be the width of the rectangle, then the diagonal (d) can be calculated using the Pythagorean theorem:

d² = L² + W²

So, the formula for the diagonal of a rectangle is:

d = √(L² + W²) = √(a² + b²)

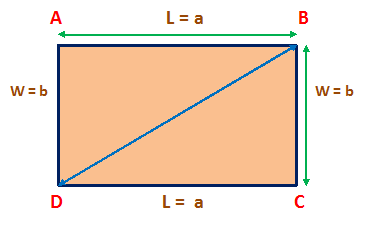
The diagonal of a rectangle is the line segment that connects the opposite corners of the rectangle. It is also the longest side of the rectangle and can be found using the Pythagorean theorem.

If the length of the rectangle is "L" and the width is "W", then the diagonal "d" can be calculated as follows:

d = √(L² + W²)

For example, if a rectangle has a length of 6 units and a width of 8 units, its diagonal can be calculated as follows:

d =  √(6²+ 8²) 

d = √(36 + 64)

d = √(100)

d = 10

Therefore, the diagonal of this rectangle is 10 units.  (Ans.)